Folding ruler roof slope scale calibration:

How to calculate or measure the scale intervals to use with a folding ruler to permit estimateing roof slope from the ground using just a folding carpenter's ruler or similar measuring instrument.

This article gives several methods for setting the scale that can be used along the horizontal portion of a folding ruler so that by bending the first two ruler segments to match the roof slope we can estimate its pitch - right from ground level.

Roof measurement methods: these articles explain various methods for measuring all roof data: roof slope or pitch, rise, run, area, and other features. This article series gives clear examples just about every possible way to figure out any or all roof dimensions and measurements expressing the roof area, width, length, slope, rise, run, and unit rise in inches per foot.

Methods of Folding Rule Inch-Scale Calibration to Read Roof Slopes & Angles

As perhaps you've see from out other roof measurement examples beginning

at ROOF MEASUREMENTS, knowing the roof slope is really handy as something we can measure up close but also from a distance, obtaining a number that plugs into simple formulas for roof area too.

At ROOF MEASURE by FOLDING RULE we demonstrate how to use a folding ruler to get a pretty good estimate of roof slope - a number that can also be used to get roof area and estimate the number of squares of roofing materials needed without ever setting foot on most roofs.

Included that article,

at FOLDING RULER to SLOPE DATA TABLE we give a table of folding ruler horizontal scale inch-numbers that correspond to different roof slopes for both shed roofs and gable roofs such as that shown in our photo above.

But how did we come up with the data table? We did not invent this idea, but unable to find a clear current explanation of the slope to ruler inch marks conversion data nor its calculation, we tested and documented the procedures explained here.

Here we describe approaches that can be used to calibrate the folding rule inch-readings that give roof slope in the procedure described above.

We can set the slope of folding ruler segments using a protractor and note where the folding ruler crosses the horizontal ruler scale: easy but you have to work with care, or we can calculate the number of inches of horizontal run for various triangles all of whom have a 6-inch hypotenuse but whose angle and thus horizontal run length change, using trigonometry.

Method 1 below, using a protractor, can be used to come up with a ruler to roof slope translation table for other folding rulers that use different scales such as centimeters.

Method 1: Angle measurement using a protractor to figure folding ruler inch-scale roof slope amounts

Shed Roof Slope or Angle Measurements Using a Folding Ruler

We use a protractor to check the angles formed by the folding rule segments with respect to the horizontal ruler base. This is a bit rough since it is difficult to place & hold the protractor in the precisely correct position.

But it's quick and you don't have to be a trigonometric expert.

To use a protractor accurately we taped a protractor image onto square-ruled paper and drew extended horizontal and vertical lines that help keep the folding ruler straight with respect to the angles being measured.

Hold the folding ruler so that its bottom scale is on or exactly parallel to the bottom horizontal line of the protractor - the 0 - 180 deg line in our photo at left.

Bend the second ruler segment (6 to 12") to the desired angle, taking care that the left edge of the ruler crosses through the origin of the protractor (where it's 90 deg. or vertical line crosses its 180 degree horizontal line).

The left edge of the ruler segment is placed on the desired angle.

In my example I've set this "roof slope" to 70 degrees.

I left the first ruler segment (0-6") bent up out of the way so that you could see my ruler's edge on the 70 degree mark of the protractor.

Remember to set the origin of the acute angle being measured accurately on the origin or zero point of the protractor (the center of the protractor's bottom scale, in line with the 90 deg. mark).

For a shed roof we then used a credit card to be sure that our vertical segment was indeed set to 90 degrees.

Below you can see that our 90 degree line (for the rise line of a shed or single slope roof) crosses the ruler's horizontal scale at 14 11 1/6".

So we know that for a single slope roof (or a right triangle) at an angle of 70 degrees, our rise line (the vertical line) crosses at 14 11/16".

Translating, if in the field we set our folding ruler with care to match a shed roof slope and then find that our rise line crosses the ruler bottom segment at 14 11/16" our roof slope is bout 70 deg.

Using this method for a range of angles, it was easy to read the folding ruler inch-scale and to make up our table converting ruler inch scale readings to roof slopes expressed in rise/run, degrees, or the Tangent value.

We also provide this TANGENTS TABLE

[Click to enlarge any image]

But when using the folding ruler to measure roof slope, because the ruler is built of fixed 6-inch segments, we are keeping the hypotenuse of our little ruler triangle fixed (at 6") and instead we vary the vertical rise and the horizontal run.

Well I'm fibbing. the hypotenuse length we are using is not exactly 6" because the 6-inch point is actually where the rivet runs right through the 12-inch mark (see my photo above) - which is in the middle of the ruler. But I prefer to make all alignments and measurements using the inside edge of the ruler triangle - somewhat shorter lines.

We don't care about this fudging as long as we are consistent, always aligning the folding rule the same way and making consistent readings on the horizontal scale.

I figure that it is more precise and also more accurate to read the inch scale along the top of the horizontal segment than to guess at the approximate center point - not an issue for single slope roofs, but at two-slope or gable roofs shown below, you will see that trying to read anywhere other than the top edge of the rule makes for trouble.

We say more about the confusion that can be wrought by 6-inch rather than 12-inch ruler segments following the protractor photos when we discuss using trigonometry to calculate roof measurements & angles.

Gable Roof Slope (two slope) or Angle Measurements Using a Folding Ruler

This method for measuring gable roof slope presumes both left and right roof slopes of the gable roof are the same.

If the roof slopes or pitch are different left and right, you just need to measure them individually using the shed roof angle measurement I just described above

To make up protractor-measured values of gable roof angles to which we bend the folding carpenter's rule we first used the protractor to set the right-hand slope and then the left hand slope. In my example at left I had also set the apex of my triangle to 90 degrees using a square. So I expect the two lower angles to each be 45 degrees - a 12" in 12" roof (with a Tangent value of 1).

Watch out: I found this double-slope folding ruler calibration approach very difficult follow accurately. A better approach to setting up the calibration scale for gable roof slopes using a folding ruler would be to follow the shed roof slope method above, then simply double the shed roof scale horizontal distance from right to left to make up a two-slope gable roof.

First set the right hand slope angle using the protractor. Remember to keep the angle origin at the protractor's center or zero point.

Then holding that folded ruler tightly to avoid disturbing its angle we set the left-hand slope using the first ruler segment.

Watch out: When using a protractor to set or check the folding ruler segment angles remember to

• Keep the bottom or horizontal ruler segment level and parallel to the bottom horizontal line (0-180 deg) of the protractor. This is where taping the protractor to square-ruled paper comes in handy.
• Keep the origin of the acute left or right folding ruler segment angles right on the protractor origin part.
• Read the angle where the folding ruler-triangle inside edges cross the marks along the protractor by looking straight down. Viewing from an angle will introduce an error.
• Read the inch scale by noting the horizontal segment inches where the left-hand folding ruler segment (corresponding to the left-hand roof slope) crosses the top of the horizontal segment.
  
  It is important to be consistent - always reading inch markings the same way to avoid an error.
• Check your angles and measurements before writing down your calibration data.

So on the left corner we want the corner of inch #1 on the first ruler segment to be in the center of the horizontal segment - thus matching lower left and right triangle corners.

Now a protractor is properly placed so that its origin is exactly where the corner of inch #1 touches the horizontal segment.

You should see that the first folding ruler segment is perfectly aligned with 45 degrees on the protractor.

Now check the left side of your triangle with the protractor to confirm that it too is reading 45 degrees.

Read the inch scale along the top of the folding ruler-triangle's bottom segment.

You could read elsewhere, like in the center of the rule or where the bottom corner of the triangle's left segment touches the rule (20 1/4") but your ruler inch conversion table to slope or roof angles has to have been made the same way you are reading your ruler.

For consistency I read the bottom segment inch scale along the top of my ruler. So showing is 19 7/8".

If you don't have a protractor, here is

a PRINTABLE PROTRACTOR [Image file].

That tells us that 19 7/8" on the top of the ruler scale is seeing a roof slope of 45 degrees (for the case of both roof surfaces having the same pitch).

Watch out: for very low slope roofs, it becomes impossible to read the slope scale along the top edge of the bottom segment of the folding ruler. You may need to make all observations and scale conversions to the outer edge of ruler segments - but that's a different table from the one we provide above.

Yes I know, if you have a roof protractor you can put it on the roof, or on the attic ceiling and read the roof slope directly as an angle or in degrees.

Folding Rule Horizontal Scale Calibration by Calculations

Method 2: Calculations using trigonometry 

or the tangent function to calculate the number of inches of run along the horizontal ruler scale that will correspond to a given roof angle for a fixed roof length. Ultimately as we explain this approach did not work for a folding ruler.

Or see TANGENTS TABLE

If you want to cut to the case read the fine print:

Watch out: for cases such as our folding ruler triangles, you are not working with a fixed horizontal unit run of 12-inches, nor an exact 6-inch roof slope length (hypotenuse of the triangle).

You will observe that the actual length of a folding ruler segment bent to follow a roof slope will lengthen as the slope is steeper and shorten as the slope is less steep. This is because the hinge point of the ruler segment is fixed and the edge of the sloping ruler segment moves closer to or further from the top of the horizontal ruler segment as you fold the ruler.

The math is easy but unfamiliar to lots of people (including me) and it's easy to mess up because

We are used to thinking of slopes as an amount of rise per unit run (vertical rise, horizontal run) while letting the roof length remain an unknown (the length of the hypotenuse of a right triangle).

But with the folding ruler roof slope method, we are always working with a triangle whose sloping sides are fixed at 6" for a gable roof, or whose single sloping side is fixed at 6" for a shed type roof. In other words we are working with triangles whose hypotenuse is always fixed at 6" but whose base length varies.

We are used to working with roof rise or slope expressed as inches of rise per foot (12") of horizontal run. But with the folding ruler slope method we work with folding ruler rise or slope for a triangle with a fixed hypotenuse of 6".

The rise can be physically measured by measuring the distance from the apex of the triangle to the horizontal base (make all measurements on the inside of the ruler-triangle), but remember that the rise you see is for a variable run, not a run fixed at 6".

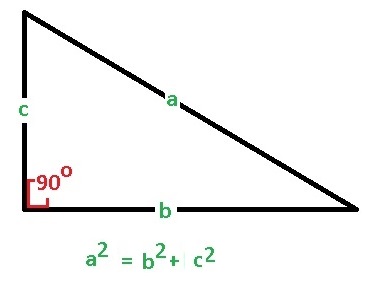
In other words, usually we think of the roof slope triangle with the horizontal run fixed at 12" and we measure either the horizontal run or we measure the length of the roof slope (they hypotenuse of the triangle) and calculate the unknown number from the familiar a² = b² +c² formula for the relationship of the 3 sides of a right triangle, where a is the roof sloe or hypotenuse of the triangle.

But here the horizontal run per roof slope (there are two slopes for a gable roof and just one slope for a shed roof) would be measured from the acute angle origin at the left or right corner of our roof triangle to the 90 degree point or to a vertical line drawn from the apex of the triangle to its base.

(That trick allows us to continue to work with right triangles to calculate ruler scale positions even when working with a 2-slope gable roof.)

For any right triangle we can write the relationships of the lengths of its sides as

a² = b² + c²

where

a = hypotenuse or the sloping roof "width" if we are measuring a building roof

c = the vertical height or the rise of the roof if we are measuring a roof

b = the horizontal run, the distance traveled horizontally as we move up (or down) the sloping or rising surface (and distance) a, achieving the ending vertical height c.

Using the folding rule we tried assuming that c was fixed at 6" and we varied a and b.

For a right triangle with the length of any two sides known can use algebra to calculate the length of the third side. Alternatively if we know the angle in degrees we can obtain the tangent value that in turn can give us the length of two unknown sides if we know the length of just one side. For example we can calculate the length of the slope a = sqrt (c² + b²)

Or we can use Tan <A = Rise / Run to compute run b by re-writing the formula as Run = Rise / Tan <A

But we found this direct calculation impossible.

Because the folding rule is hinged at the center of each segment, as we change the angle of segments with respect to one another, the lengths of the inner triangle segments changes.

This awful development led to giving up on calculating the horizontal index values for a folding rule measuring roof slope.

It is possible, by considering the width of the ruler segments, the hinge pin diameter, and the amount of change of length of each segment as it hinges over another segment to any position other than straight-out. But really! Perhaps an interested reader will give us that formula.

...

Continue reading at ROOF CALCULATIONS SUMMARY or select a topic from the closely-related articles below, or see the complete ARTICLE INDEX.

Or see these

Recommended Articles

• 6-8-10 RULE to SQUARE UP ANY STRUCTURE
• ANGLE FINDER LEVEL
• ARCTANGENT CALCULATES ROOF / STAIR ANGLE
• CALCULATE RISE & RUN from SLOPE
• SQUARE PLUMB & LEVEL A STRUCTURE
• FOLDING RULER ROOF SLOPE CALIBRATION
• FRAMING SQUARE-UP 6-8-10 RULE
• FRAMING SQUARE USER'S GUIDE
• FRAMING TRIANGLES & CALCULATIONS
• FROGS HEAD SLOPE MEASUREMENT
• HOPPUS MEASURER
• PRINTABLE PROTRACTOR [Image file]
• RAMP SLOPE or PITCH
• ROOF AREA CALCULATIONS
• ROOF MEASUREMENTS
  • ROOF MEASURE ON ROOF
  • ROOF MEASURE INDOORS
  • ROOF MEASURE at GABLE END
  • FRAMING SQUARE USER'S GUIDE
  • ROOF MEASURE FROM GROUND
  • ROOF MEASURE by FOLDING RULE
  • ROOF CALCULATIONS SUMMARY
  • ROOF ESTIMATES by FLAT PROJECTION
• ROOF SLOPE CALCULATIONS
• STAIR RISE & RUN CALCULATIONS

Suggested citation for this web page

FOLDING RULER ROOF SLOPE CALIBRATION at InspectApedia.com - online encyclopedia of building & environmental inspection, testing, diagnosis, repair, & problem prevention advice.

Or see this

INDEX to RELATED ARTICLES: ARTICLE INDEX to BUILDING ROOFING

Or use the SEARCH BOX found below to Ask a Question or Search InspectApedia

Ask a Question or Search InspectApedia

Try the search box just below, or if you prefer, post a question or comment in the Comments box below and we will respond promptly.

Note: appearance of your Comment below may be delayed: if your comment contains an image, photograph, web link, or text that looks to the software as if it might be a web link, your posting will appear after it has been approved by a moderator. Apologies for the delay.

Only one image can be added per comment but you can post as many comments, and therefore images, as you like.
You will not receive a notification when a response to your question has been posted.
Please bookmark this page to make it easy for you to check back for our response.

---

 

IF above you see "Comment Form is loading comments..." then COMMENT BOX - countable.ca / bawkbox.com IS NOT WORKING.

In any case you are welcome to send an email directly to us at InspectApedia.com at editor@inspectApedia.com

We'll reply to you directly. Please help us help you by noting, in your email, the URL of the InspectApedia page where you wanted to comment.

Citations & References

In addition to any citations in the article above, a full list is available on request.

---

ADVERTISEMENT

---