Framing Square Tables, Uses, Instructions
Framing Square Tables, Uses, Instructions
Layouts, Measurements & Cuts Using a Framing Square & Its Tables
Roof rise, run, rafter cuts, braces, or stair stringer layout using a framing square
- POST a QUESTION or COMMENT about types of roofing materials, installation, inspection, diagnosis, repair, maintenance, & warranties
How to use a framing square.
A framing square is a lot more than a simple square-cut saw guide. This simple device is crammed with tables, data and tricks that allow a carpenter to lay out roof rafters, stairs, or other building features.
A carpenter's framing square includes tables stamped right into the tool itself. This article explains how to make quick use of a framing square and its imprinted data to get some basic roof measurement data like roof pitch or slope, rafter lengths, and end cuts, stair stringer cuts, lengths of braces and other construction measurements.
Roof measurement methods: these articles explain various methods for measuring all roof data: roof slope or pitch, rise, run, area, and other features. This article series gives clear examples just about every possible way to figure out any or all roof dimensions and measurements expressing the roof area, width, length, slope, rise, run, and unit rise in inches per foot.
InspectAPedia tolerates no conflicts of interest. We have no relationship with advertisers, products, or services discussed at this website.
Guide to Using a Carpenter's Square / Framing Square
 The standard two-foot framing square, also referred to as a "carpenter's square" and historically as a "steel square" is an L-shaped tool that is used to mark angles for cuts used in building framing, particularly roof rafters, stair stringers, and many other cuts or angles other than 90°.
The standard two-foot framing square, also referred to as a "carpenter's square" and historically as a "steel square" is an L-shaped tool that is used to mark angles for cuts used in building framing, particularly roof rafters, stair stringers, and many other cuts or angles other than 90°.
On both the long and short arms of the framing square are marked various framing tables giving rafter lengths, roof slopes and the proper angle of cuts for various roofing connections such as a rafter end abutting the ridge board, the birds' mouth cut at the rafter segment that rests atop a wall plate, hip and valley rafter cuts and other information.
Here we explain just how to use a framing square and its etched-on tables to figure out roof slope & rafter lengths, rafter cuts, brace lengths & cuts, stair stringer layout & cuts and other construction & framing layouts & saw cuts.
We start by defining the parts of the square, then we list each of the tables found on the carpenter's square and we explain what the table is for.
That list includes links to detailed procedures for using each of the tables on the square.
Special tables give the number of joists, studs, or rafters in a floor, ceiling, wall or roof of given dimension. Another clever table allows you to accurately frame complex structures such as an octagon
Other tables include conversions of inches of rise/run to slope in degrees, conversion of fractions of an inch (1/32, 1/16, 1/8 etc. ) to decimal fractions, conversion of inches to decimal fractions of a foot, numbers of joists, studs, rafters, and even pilot hole size recommendations for wood screws.
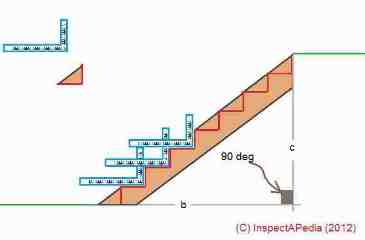
Our sketch above illustrates just one of many uses of the carpenter's square: to lay-out a stair stringer, steps and step rise.
[Click to enlarge any image]
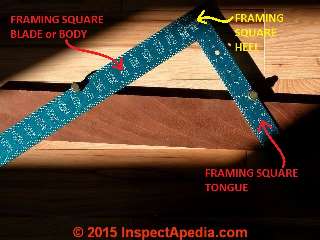
Definition of the Parts of a Framing Square: blade, tongue, heel, front, back
The parts and faces of the framing square labelled in the framing square photograph above, and are referred to as follows:
- The framing square BLADE or body:
this is the wider and longer arm of the "L", normally 2" in width and 24" long.
The "fatter" and "longer" length of the framing square is its blade.
[Click to enlarge any image]
- The framing square TONGUE:
this is the shorter and more narrow arm of the "L", normally 1 1/2 " in width and 16" long.
The "skinny" and "shorter" length of the framing square is its tongue.
- The framing square HEEL:
is the point where the two arms of the framing square meet.
In our framing square photo above the framing square heel is indicated by the yellow arrow.
Below we take a closer look at the heel face and the back of the heel of the framing square.
- The framing square FRONT or face
is the side of the square you will see if you look at the square while holding its longer arm, the tongue, horizontally with the shorter arm, the tongue to your right and pointing down.
When held in this position, the wider longer blade or body arm is pointing to my left and shows me a table of common rafter lengths per foot of run, hip or valley rafter lengths per foot run. Below our photo shows the front or face view of a framing square.
The face of the framing square, by custom, is the side imprinted with the manufacturer's name and perhaps a patent identification. More useful, on the face of the framing square blade you'll find rafter framing tables.
- The framing square BACK
is the side of the framing square that you will see if you look at the square while holding its longer arm, the tongue horizontally with the shorter arm, the tongue, to your left and pointing down, as cute little Fala is demonstrating in the framing square back photo below.
Fala's little red tongue is licking the TONGUE of the framing square BACK.
When held in this position the wider longer blade or body arm is pointing to the right.
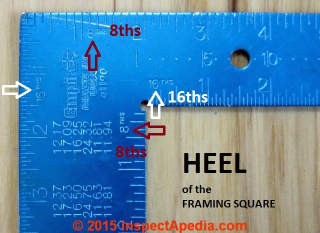
A Closer Look at the Framing Square Heel
Below our photo shows the front or face side of the heel of a framing square. You'll see that the manufacturer's name (Empire) and the framing square model number (e1190) appear on the framing square's heel face.
Watch out: the measurement scales along various edges of the framing square are not all in the same units.
We will discuss the inch-divisions shown on the heel face of the framing square in just a bit further along in this article
at FRAMING SQUARE DIVISION WARNINGS 8ths 10ths 12ths & 16ths.
List of the Tables Found on a Framing Square
 Here we'll list all of the tables found on a decent quality framing square, then we'll explain how each of them is used.
Here we'll list all of the tables found on a decent quality framing square, then we'll explain how each of them is used.
6 Rafter Tables Found on the FRONT of a Framing Square BLADE
Seen from the top edge of the framing square blade then moving downwards, on the front of the blade when you hold the framing square with the tongue or shorter arm to your right and pointing down you'll see inches marked in 16ths on the blade top edge, then the tables we give below, and finally inches marked in 8ths on the bottom edge of the blade.
- COMMON RAFTER LENGTH PER FOOT OF RUN - this is the top line in the rafter length tables
- HIP OR VALLEY RAFTER LENGTH PER FOOT OF RUN
- DIFF IN LENGTH OF JACKS 16 INCH CENTERS
- DIFF IN LENGTH OF JACKS 24 INCH CENTERS
- SIDE CUT LENGTH OF JACK RAFTERS
- SIDE CUT OF HIP RAFTER OR VALLEY RAFTER
All of the tables listed above are explained in detail at FRAMING SQUARE RAFTER LENGTHS - explaining each of the 6 tables on the framing square's blade front
Above: an example of one of the rafter length data columns explained in the article cited just above.
Octagon Table Found on the FRONT of a Framing Square TONGUE
When you hold the framing square with the tongue or shorter arm to your right and pointing down, on the outer edge of the framing square tongue are inches marked in 8ths, then the table I cite below, and finally inches marked in 16ths on the inner edge of the tongue.
Details about reading and using the octagon table on a framing square are found at
FRAMING SQUARE OCTAGON TABLE in multiples of 5 (located in the middle of the tongue)
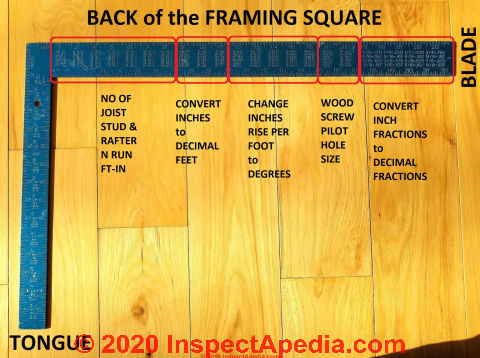
6 Tables Found on the BACK of a Framing Square BLADE
These tables are on the framing square back, on its long arm or "blade"
- Blade Back, under inch-marks 2-8: explained in detail at FRAMING SQUARE N JOISTS RAFTERS N RUN FT-IN - the start of this table is shown above
- Blade Back, under inch-marks 9 11: explained in detail at CONVERT INCHES to DECIMAL FRACTIONS of a FOOT
- Blade Back, under inch-marks 12-16: explained in detail at CONVERT INCHES of RISE per FOOT to DEGREES of SLOPE
- Blade Back, under inch-marks 17-18: explained in detail at WOOD SCREW PILOT HOLES
- Blade Back, under inch-marks 19-23: explained in detail at CONVERT DECIMAL FRACTIONS TO INCHES
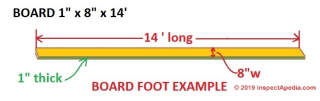
- FRAMING SQUARE ESSEX BOARD MEASURE - older squares, estimate number of board feet in a quantity of lumber of given dimensions
Also see HOPPUS MEASURER for the history of board feet.
Brace Triangle Table on the Framing BACK of the Framing Square TONGUE
This table is also called the "Diagonal Scale" table.
This table shows us the length of the brace arms needed for diagonal braces used, for example, to stabilize & support posts used in timber frame construction, or in bracing the posts of a deck or porch.
The framing square Brace Triangle Table , also referred to as the Brace Rule - is discussed in detail
Other Framing Square Tutorials
- FRAMING SQUARE DIVISION WARNINGS - 16ths, 12ths, 10ths, 8ths on various scales: watch out!
- FRAMING SQUARE COLOR & MATERIALS - black gets hot
- FRAMING SQUARE DETERMINES ROOF SLOPE - neat tricks
- FRAMING SQUARE for STAIR BUILDING - layout stair stringers, steps
- FRAMING SQUARE TRUING PROCEDURE - fix the framing square
- FRAMING SQUARE HOW-TO MANUALS - downloads
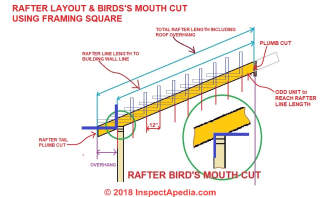
- FRAMING SQUARE for BIRDS MOUTH CUT - how to notch rafters at the top plate
- FRAMING SQUARE ESSEX BOARD MEASURE
-
FRAMING SQUARE ESSEX BOARD MEASURE found on the back of some older framing square blades gives the number of board feet in lumber of any dimension.
- FRAMING SQUARE-UP 6-8-10 RULE - really easy way to make sure your framed structure is squared-up.
How to Use the Tables on the BACK of a Framing Square
The Number of Joists, Studs, Rafters N Run Ft-In Table on a Framing Square Blade Back
Found beginning under the 2-inch column on the back of a framing square blade and extending to below the 8-inch column of data is a table titled
NO OF
JOIST
STUD &
RAFTER
N RUN
FT-IN
This table gives the number of framing members N in a floor, ceiling, wall or roof of width (or "run") W.
Complete details about this table and how to use it are found at
FRAMING SQUARE N JOISTS RAFTERS N RUN FT-IN
Convert Inches to Decimal Fractions of a Foot using the Framing Square
Found under the 9-11 inch columns on the framing square blade back, this framing square table converts inch measurements into decimal fractions of a foot.
For example:
- 1/2" = .04 ft.
- 6" = .50 ft.
- 12" = 1.0 ft.
Change of Inches of Rise per Foot into Degrees of Slope on a Framing Square Blade Back
Found under the 12-16 inch columns on the framing square blade back, this table helps you convert inches of rise to slope expressed in degrees.
For example:
- 6" of rise per foot of run = 26.50 degrees of slope
- 10" of rise per foot of run = 40 degrees of slope
- 18" of rise per foot of run = 56.25 degrees of slope
- 24" of rise per foot of run = 63.50 degrees of slope
See ROOF SLOPE TABLE, TYPES, WALKABILITY for a detailed table of roof slopes in rise & degrees as well as comments about roof slope walkability
Table of Wood Screw Pilot Hole Sizes on the Framing Square Blade Back
Found under the17-19 inch columns on the framing square blade back, Empire includes a table of recommended pilot hole diameter for wood screws of various sizes.
For example, in the table shown above, if you are using a #10 wood screw the pilot hole should be 0.109"
Convert Inch Fractions (32nds, 16ths, 8ths, 4ths) to Decimal Fractions on the Framing Square
Found under the 19-23 inch columns on the framing square blade back, near the right end of the back of our framing square blade this table converts common inch-fractions from 1/32" to 15/16" into decimal fractions.
A savvy carpenter will use this table given on the right end of the back side of the framing square body to find common (close enough) conversions of fractions of an inch to 8ths, 16ths, or 32ds. That table is shown just below.
Examples:
- 1/32" = 0.31"
- 1/16" = 0.62"
- 5/8" = 0.625"
Let's use the decimal to inch conversion table above to find the length of our brace hypotenuse in the 33 33 46.67 example given above.
We want to convert 46.67" to something in eights or sixteenths of an inch. Look into the table and find the decimal fraction closest to .67.
I see that the entry for 5/8" = .625" and the next bigger entry is for 11/16" = .687". Without a lot of thought I think figure that .687 is the closest number to my target of .67" so I'll measure my cut to 46 11/16". Let's see just how close this table gets us to spot-on by calculating the numbers instead of using the table above.
Whip out your cellphone, turn on its calculator and multiply the number of sixteenths in an inch (that's 16 of course) by .67 (the fraction of an inch given in the table) and you'll get 10.72 sixteenths.
That tells me that if I'm measuring my cut to the nearest 16th of an inch I'll measure out 46" and another 10 1/2 sixteenths inches or 46 10/16" plus a hair as we say in the trade.
And you can see that the table gave us 46 11/16" while the calculation gave us 46 10/16" plus a hair. In other words either approach gives us something between 46 5/8 (that's the same as 46 10/16) inches and 46 11/16 inches.
What's nice about these brace length numbers is that if you measure the hypotenuse length carefully to match the numbers in the brace table you can just slap your speed-square or try-square onto the length measurement point and with your square set at 45 degrees, mark your cut.
For my example, your triangular layout of the brace, measured on the outermost edge of the bracing member, will be 33" on the two short sides and 46" on the long side.
Warning About the Different Inch-Divisions on the Framing Square
Why do we care about identifying the "face" or the "back" of the framing square? Because the two arms and four sides of them contain different tables of saw cut information that some texts will identify by their location on the framing square.
Watch out: you can get fouled up by different subdivisions of the inch markings on the face and back of a framing square. Remember to check the divisions as the 12ths and 10ths below can lead you to make a measuring or saw cut mistake.
Some texts say "always make your measurements using the outside edges of the framing square so that you won't get fouled-up. That advice does not work. For example, the front outside edges of the framing square measure 16ths or 8ths of an inch, depending on which blade you use, but the back outside edges of a framing square are marked in 12ths.
Our photo above shows the inch divisions on the back of a framing square:
- On the back of a framing square along the outer edges of both the blade and tongue the inches are divided into 12ths (yellow arrows).
- On the back of a framing square along the inner edge of the tongue (the shorter arm) inches are divided into 10ths (green arrow).
- On the back of a framing square along the inner edge of the blade, inches are divided into 16ths (white arrow).
- On the front of a framing square along the outer edge of the blade (the longer arm) inches are divided into 16ths
- On the front of a framing square along the inner edge of the blade inches are divided into 8ths.
- On the front of a framing square along the outer edge of the tongue (the shorter arm) inches are divided into 8ths
- On the front of a framing square along the inner edge of the tongue inches are divided into 16ths.
Framing square color and metal - do they matter?
 Early framing squares were made of hardwood and carefully trued then braced into a right angle.
Early framing squares were made of hardwood and carefully trued then braced into a right angle.
Modern carpenters have used steel squares and more recently aluminum framing squares for decades.
The traditional steel framing square was black while newer framing squares are often made of aluminum. Who cares what color or metal comprise your framing square? You might.
My first framing square was made of steel and was black in color with white numbers and lines.
In the photo of my old hand tools (above), the red arrow points to this framing square while the green arrow points to an aluminum speed square whose added thickness forms a terrific saw guide.
More about these hand tools is
at DECK BUILDING & CARPENTRY TOOLS.
My old black steel framing square was easy to read in various lighting conditions, was very resistant to gouges from my rock knife when I used the square to make cuts in drywall or to make cuts in lumber as one might do to avoid chipping up wood when cross-cutting with a power saw.
This black square also got hot as hell when left flat in bright sunlight. And as the finish wore off of this tool, if I did not use it often enough it was prone to rust.
My current framing square, made by Empire Level, is made of light blue aluminum. The numbers and lines are of the same color and are quite a bit harder to read in some light conditions.
This square is also easily gouged and scarred when in use. It is a bit lighter and a bit cooler in the hot sun. I miss my old square but truth is, I had allowed the old one to get a bit rusty.
Framing square number legibility improvement tip:
As the numbers and line markings on your framing square are recessed, having been stamped into the metal of its body, you can improve the legibility of a monotonic framing square (for you carpenters that means it's all the same color), by painting the whole square surface with white latex paint.
Right after you've made a horrible white painted mess of the square's surface, wipe it off before the paint dries.
You'll see that some white paint will remain in the recessed numbers and grooves of the tool. The manufacturer could have done this too, but then they'd have to charge you more for the tool.
Using the Framing Square to Build Stairs
Separately at DECK STAIR BUILDING DETAILS we explain in detail how a framing square is used to layout rise and run on the stair stringers when building stairs.
Roof Framing Example 5: Location of the Bird's Mouth Cutout in the Rafter
This discussion now has its own page at FRAMING SQUARE for BIRDS MOUTH CUT
How to Obtain Roof Pitch or Slope by Using the Rafter Tables on a Framing Square
This discussion got promoted to its very own web page
at FRAMING SQUARE DETERMINES ROOF SLOPE
Framing Square History, References Articles & How-To Manuals
This section has moved to
At the end of this article atReferences or Citations you'll find more texts and articles on using a framing square and written by other experts.
...
Continue reading at FRAMING SQUARE RAFTER LENGTHS or select a topic from the closely-related articles below, or see the complete ARTICLE INDEX.
Or see FRAMING SQUARE USER'S GUIDE FAQs - Questions & answers posted originally on this page
Or see these
Building & Roof Measurement Articles
- 6-8-10 RULE to SQUARE UP ANY STRUCTURE
- ANGLE FINDER LEVEL
- ARCTANGENT CALCULATES ROOF / STAIR ANGLE
- CALCULATE RISE & RUN from SLOPE
- DECK LEVEL PLUMB SQUARE-UP 6-8-10 RULE
- FOLDING RULER ROOF SLOPE CALIBRATION
- FRAMING SQUARE USER'S GUIDE
- FRAMING SQUARE PARTS DEFINED
- FRAMING SQUARE TABLES LIST
- FRAMING SQUARE RAFTER LENGTHS
- FRAMING SQUARE for BIRDS MOUTH CUT
- FRAMING SQUARE OCTAGON TABLE
- FRAMING SQUARE BACK TABLES
- FRAMING SQUARE BRACE TABLE
- FRAMING SQUARE N JOISTS RAFTERS N RUN FT-IN
- FRAMING SQUARE ESSEX BOARD MEASURE
- FRAMING SQUARE DIVISION WARNINGS
- FRAMING SQUARE COLOR & MATERIALS
- FRAMING SQUARE DETERMINES ROOF SLOPE
- FRAMING SQUARE for STAIR BUILDING
- FRAMING SQUARE-UP 6-8-10 RULE
- FRAMING SQUARE TRUING PROCEDURE
- FRAMING SQUARE HOW-TO MANUALS
- FRAMING TRIANGLES & CALCULATIONS
- FROGS HEAD SLOPE MEASUREMENT
- HOPPUS MEASURER
- ROOF FRAMING TIES & BEAMS
- ROOF MEASUREMENTS
- ROOF SLOPE CALCULATIONS
- ROOF SLOPE TABLE, TYPES, WALKABILITY
- STAIR RISE & RUN CALCULATIONS
Suggested citation for this web page
FRAMING SQUARE USER'S GUIDE at InspectApedia.com - online encyclopedia of building & environmental inspection, testing, diagnosis, repair, & problem prevention advice.
Or see this
INDEX to RELATED ARTICLES: ARTICLE INDEX to BUILDING ROOFING
Or use the SEARCH BOX found below to Ask a Question or Search InspectApedia
Ask a Question or Search InspectApedia
Try the search box just below, or if you prefer, post a question or comment in the Comments box below and we will respond promptly.
Search the InspectApedia website
Note: appearance of your Comment below may be delayed: if your comment contains an image, photograph, web link, or text that looks to the software as if it might be a web link, your posting will appear after it has been approved by a moderator. Apologies for the delay.
Only one image can be added per comment but you can post as many comments, and therefore images, as you like.
You will not receive a notification when a response to your question has been posted.
Please bookmark this page to make it easy for you to check back for our response.
Our Comment Box is provided by Countable Web Productions countable.ca
Citations & References
In addition to any citations in the article above, a full list is available on request.
- Spence, William P. (2000). Constructing Staircases Balustrades & Landings. Sterling Publishing. ISBN 0-8069-8101-6.
- [1] "How to Measure Angles with a Ruler", South Dakota School of Mines and Technology, Website: http://www.mcs.sdsmt.edu, http://www.mcs.sdsmt.edu/tkowalsk/portfolio/downloads/pub_HowToMeasureAngles.pdf retrieved 10/26/2013, copy on file.
- "Choosing Roofing," Jefferson Kolle, January 1995, No. 92, Fine Homebuilding, Taunton Press, 63 S. Main St., PO Box 5506, Newton CT 06470 - 800-888-8286 - see http://www.taunton.com/FineHomebuilding/ for the magazine's website and for subscription information.
- Our recommended books about building & mechanical systems design, inspection, problem diagnosis, and repair, and about indoor environment and IAQ testing, diagnosis, and cleanup are at the InspectAPedia Bookstore. Also see our Book Reviews - InspectAPedia.
- Best Practices Guide to Residential Construction, by Steven Bliss. John Wiley & Sons, 2006. ISBN-10: 0471648361, ISBN-13: 978-0471648369, Hardcover: 320 pages, available from Amazon.com and also Wiley.com. See our book review of this publication.
- Decks and Porches, the JLC Guide to, Best Practices for Outdoor Spaces, Steve Bliss (Editor), The Journal of Light Construction, Williston VT, 2010 ISBN 10: 1-928580-42-4, ISBN 13: 978-1-928580-42-3, available from Amazon.com
- In addition to citations & references found in this article, see the research citations given at the end of the related articles found at our suggested
CONTINUE READING or RECOMMENDED ARTICLES.
- Carson, Dunlop & Associates Ltd., 120 Carlton Street Suite 407, Toronto ON M5A 4K2. Tel: (416) 964-9415 1-800-268-7070 Email: info@carsondunlop.com. Alan Carson is a past president of ASHI, the American Society of Home Inspectors.
Thanks to Alan Carson and Bob Dunlop, for permission for InspectAPedia to use text excerpts from The HOME REFERENCE BOOK - the Encyclopedia of Homes and to use illustrations from The ILLUSTRATED HOME .
Carson Dunlop Associates provides extensive home inspection education and report writing material. In gratitude we provide links to tsome Carson Dunlop Associates products and services.